Understanding Frames of Reference

Often, it is believed that in the realm of special relativity, the speed of light remains unchanged across all frames of reference. This means that no matter how fast an observer is moving, they will measure the light speed as the same. This concept has significant implications, such as the helicity of photons being an invariant property. However, it’s crucial to note that light's speed can vary depending on the chosen frame of reference. In fact, one can select a frame where light travels at an astonishing speed of one quadrillion meters per second. Let's delve into why this occurs.

A "frame of reference" essentially refers to a coordinate system in which we can define physical variables. For instance, imagine a coordinate system where you are stationary at the origin, observing a car moving to the right at 5 meters per second. Alternatively, we can establish a new coordinate system that moves alongside the car, placing it at the origin. In this new system, you would appear to be moving left at 5 meters per second while the car remains still.

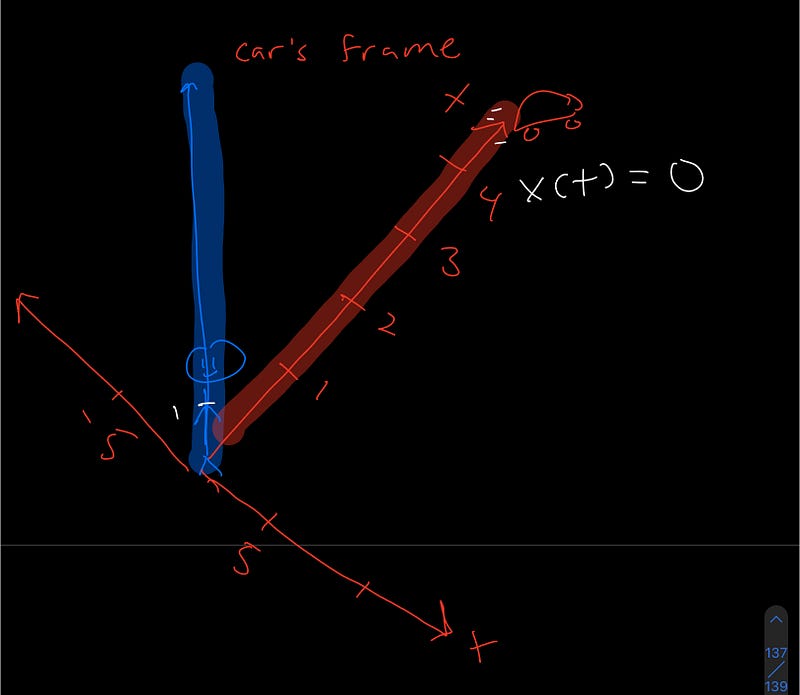
Types of Frames of Reference

There are two main categories of frames: inertial and non-inertial. While some define inertial frames as those in constant motion and non-inertial frames as those experiencing acceleration, this distinction can be misleading. Non-inertial frames perceive themselves as having zero acceleration. In essence, all frames consider themselves at the origin and do not detect their own motion. A more precise definition of an inertial frame is one that follows a geodesic, or the straightest path possible through spacetime. For instance, if you draw a great circle on a basketball's surface, you would be tracing a geodesic. Non-inertial frames, conversely, do not adhere to these geodesics. The identification of geodesics versus non-geodesics is determined by a “metric,” which is universally acknowledged by all observers. Although the appearance of geodesics may vary across different frames, all observers will agree on which paths are geodesics.

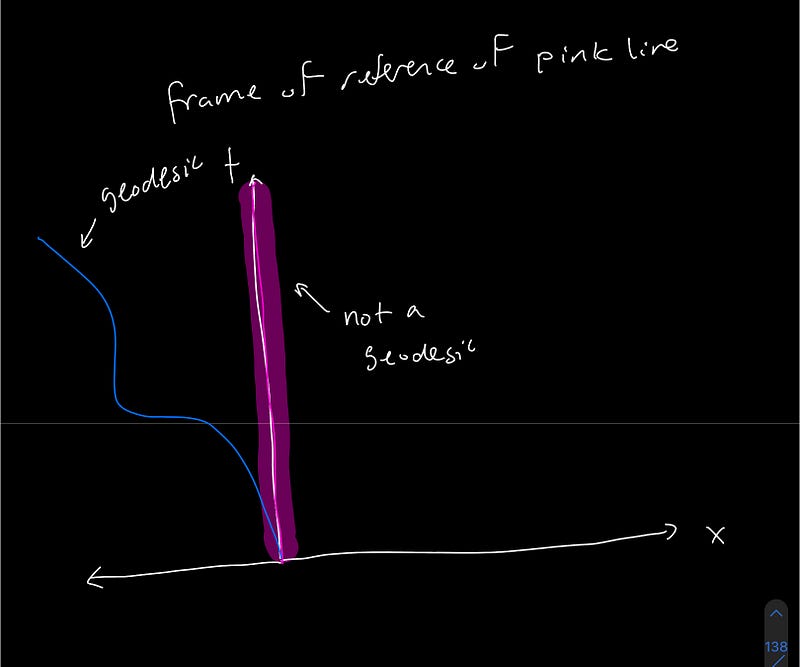
The Complexity of Light Speed

Now that we grasp the concept of frames of reference, we can explore the question of light's constancy. While special relativity presupposes that light speed is uniform across all inertial frames, it allows for variations in non-inertial frames. Observers in accelerating frames (from the viewpoint of an inertial system) will be in non-inertial frames. These non-inertial frames encounter additional forces, known as inertial forces (like the Coriolis effect). In the realm of special relativity, non-inertial observers can perceive event horizons behind them. This phenomenon occurs because certain light beams emitted may not catch up to an accelerating object, resulting in a part of the universe being "obscured" from view—a characteristic feature of a horizon (technically referred to as a rindler horizon, yet locally similar to an event horizon).

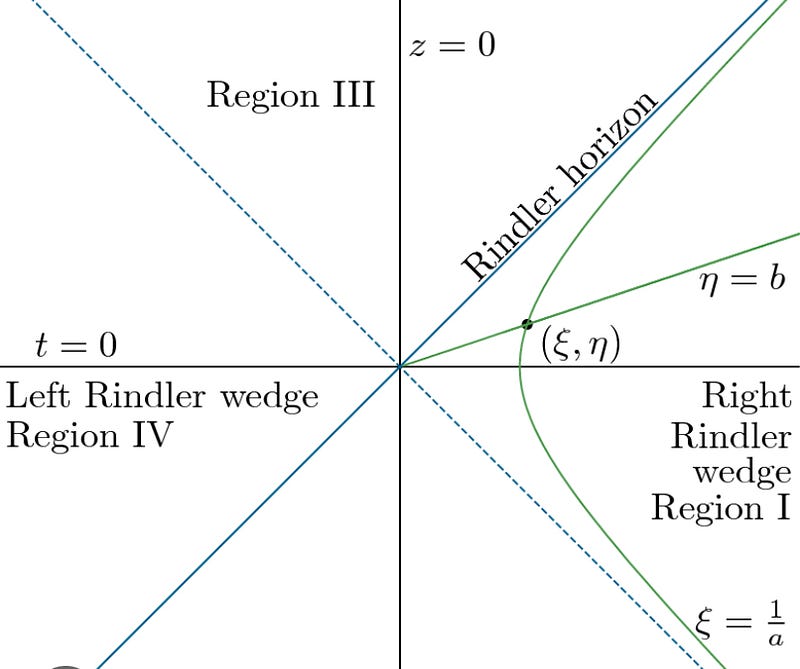
Only non-inertial frames will detect a rindler horizon. Objects approaching this horizon will appear to slow down due to time dilation, including light. Consequently, an accelerating observer might measure the speed of light as less than the standard value of c (300,000,000 m/s) due to this horizon. Notably, as light nears the horizon, the accelerating observer could register its speed as zero. Conversely, light ahead of the observer may seem to travel faster than c, with no upper limit; they could observe light moving at a trillion meters per second if it is sufficiently distant. This scenario is somewhat analogous to a "white hole," which repels everything around it, but in this case pertains to photons.

General Relativity and Light's Behavior

So, what about general relativity? Does light slow down due to gravity, even in inertial frames? The answer is affirmative. A free-falling inertial observer within the framework of general relativity will perceive the speed of light as different from c due to spacetime curvature causing time dilation. This principle holds for non-inertial observers as well. In fact, in general relativity, the speed of light can vary based on location without the necessity of a horizon, although introducing a horizon for the sake of discussion would again show that light approaches a speed of zero as it nears an event horizon from an external observer's perspective.

In conclusion, the speed of light is universally constant only when gravity and acceleration are absent. When these factors come into play, observers can measure differing light speeds. It is crucial to recognize that while the local speed of light will always measure as c—meaning you will always gauge the speed of a light beam close to you as c, regardless of your movement—its speed can vary significantly when observed from a distance. Interestingly, observers in accelerating frames may also perceive additional photons where stationary observers detect none. For further exploration on this topic, check out my channel, Fermion Physics, where I will soon demonstrate a related calculation.

The first video, "What If The Speed of Light is NOT CONSTANT?" delves into the nuances of light speed across various frames of reference and its implications in modern physics.

In the second video, "The Speed of Light is NOT Fundamental. But THIS is," the discussion shifts to the foundational aspects of light speed and its critical role in the understanding of the universe.